Derivative of Volume of a Cylinder With Respect to Radius
The rate of increase of the radius is dr dt. Choose the correct answer below.
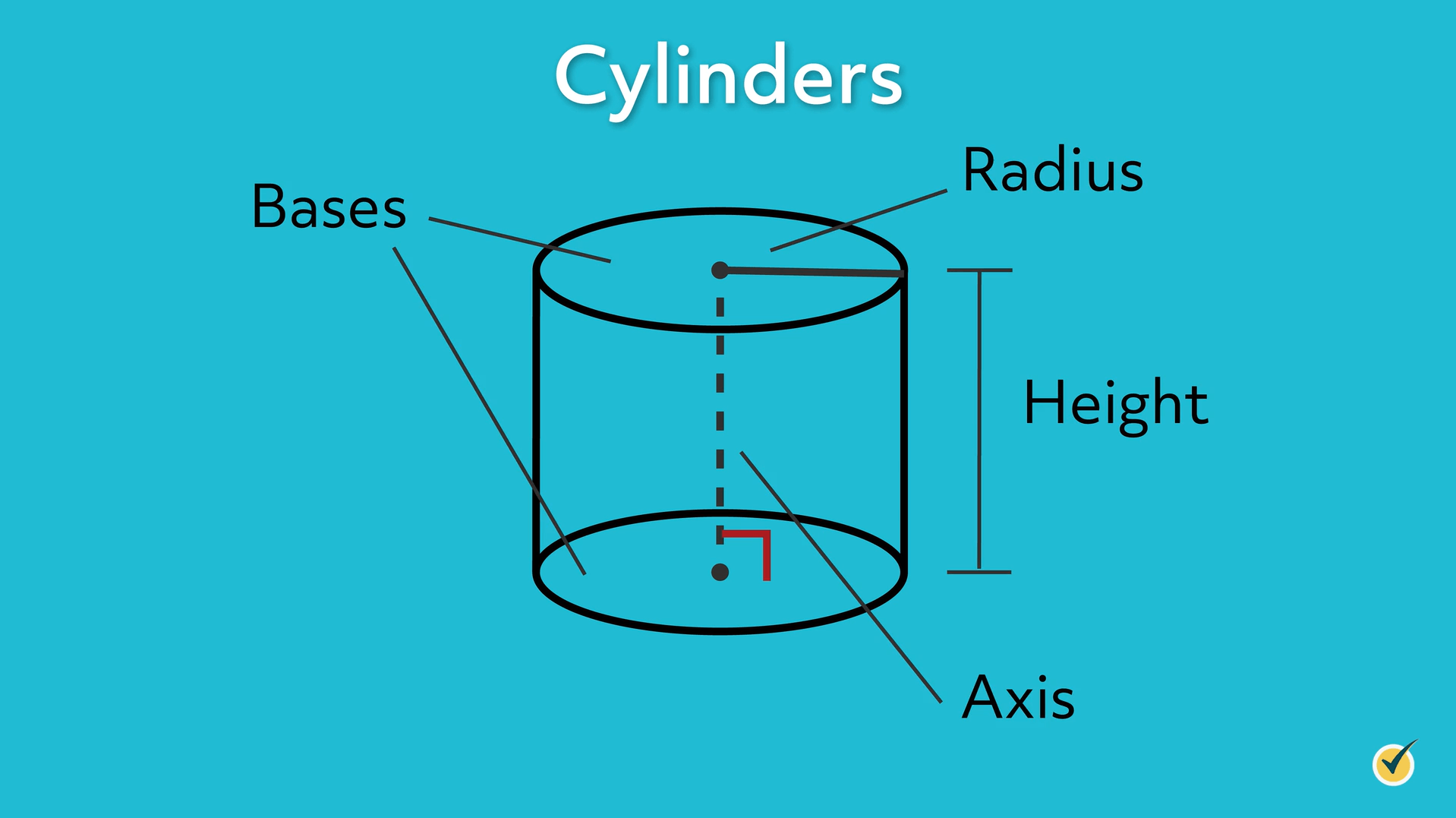
Volume And Surface Area Of A Right Circular Cylinder Video Practice
Is the volume an increasing or decreasing function o.
. Partial derivatives Find the first partial derivatives of the following functions. A cylinder uh with respect to radius when the height of the cylinder is equal to the radius. The volume of a right circular cylinder with radius r and height his VArh.
V pi r2 h Suppose you need to describe how the volume changes in response to varying just the height while keeping the radius constant. Its radius and its height. The volume cylinder is fixed at a value of V.
The derivative of r 2 with respect to r is 2r and π and h are constants It says as only the radius changes by the tiniest amount the volume changes by 2 π rh It is like we add a skin with a circles circumference 2 π r and a height of h. According to the second derivative test the volume when r 23 05 R is maximum. The volume of a right circular cylinder with radius r and height h is V pr2 h.
Find the rate of change of the volume of a sphere with respect to its surface area when the radius is varying. Find the rate of change of the total surface area of a cylinder of radius r and height h when the radius varies. So the equation for a volume for the volume of the cylinder is V equals hi R squared H.
In this problem the volume and the radius are both functions of the time t. Thus the rectangles area is 2πr h. This is the circumference of the circle and is 2πr.
The volume is a. What is the derivative of the equation for volume with respect to. FHx yLy8 2 x6 2 x y 9.
B Find the rate of change of A with respect to r if h remains constant. We know from geometry that the volume of a cylinder is found by using the formula V πr2h. Of change are derivatives.
Av The volume is a decreasing. A Find the rate of change of A with respect to h if r remains constant. Is the volume an increasing or decreasing function of the radius at a fixed height assume r 0 and h 0.
Secondly we know π is a constant and our h 55 inches dh dt 1 inchmin. But in this case we have H. The volume of a right circular cylinder with radius r and height his VArh.
The width is the height h of the cylinder and the length is the distance around the end circles. Is the volume an increasing or decreasing function of the radius at a fixed height assume r0 and h 0. The volume of a right circular cylinder with radius r and height h is V Trh.
You would like to determine the maximum volume that you can contain in a cylinder that costs less than 4. For each part of 2 use proper mathematical notation and give the units. F h π r 2 1 π r 2.
MathfracdVdhpi r2math mathfracdVdr2 pi r hmath. Consider a sphere of radius r where you want to increase the volume very slightly. Oscillations Redox Reactions Limits and Derivatives Motion in a Plane Mechanical Properties of Fluids.
Find how fast the radius of the cylinder changes with respect ti the height of the cylinder when the radius and. The volume of paint youve added to the sphere is dVareadr. Therefore the maximum weight of this cylinder is.
Is equal to our the height is the same as the radius here. The rate of increase of the volume with respect to time is the derivative dV dt. The total surface area of a right circular cylinder is given by the formula A 2pir r h where r is the radius and h is the height.
Integrate both sides which acts like adding infinitely many tiny layers. Express the volume of a right circular cylinder as a function of two variables. To calculate the derivative of anything you need to specify the variable that is changing.
Show that the rate of change of the volume of the cylinder with respect to its radius is the product of its circumference multiplied by its height. Derivative both side of equation 9 gives the second derivative of volume with respect to radius. The volume of a cylinder that is r inches in diameter and h inches in height is given by rh y2h in.
First I want it to made apparently clear that we are finding the rate of volume or dV dt. Choose the correct answer below. 7 Find the second.
FHx yL3 x2 y 2 8. Thus r t 05 and r t 05525 m where r t is the radius of cones circular base when the water is 5 m high. Combining these parts we get the final formula.
π is Pi approximately 3142 r is the radius of the cylinder h height of the cylinder. GHx yLcos 2 xy 10. 7 Find the second derivative of volume with respect to the radius.
7 Find the first derivative. The volume of a cylinder is mathVpi r2 hmath Here are some possiblilities. Thus I use the formula of the cone volume V t pi3 r t2h t Then I find the derivative of V t and using the fact that V t 8 r t 25 r t 05 I solve the equation and find h t.
The volume of a cylinder can be described as a function of its height h and its radius r. OV The volume is an increasing function because the partial derivative of V with respect to r is given by 2 ch OB. You can do this by adding a layer of paint covering the entire surface area with a tiny thickness dr.
We now find a derivative of our. For the partial derivative with respect to h we hold r constant. Thirdly our r 2 inches since D r 2 or 4 2.
Is the volume an increasing or decreasing function of the radius at a fixed height assume r 0 and h0. 7 Find the first derivative of volume with respect to the radius b. It has been determined that when the value of the first derivative of volume is 0 the value of the second derivative is less than 0.

Finding Volume Of Cylinders Finding Volume Geometry Lessons Volume Of A Cylinder

Formula Volume Of Cylinder Explained With Pictures And Examples The Formula For Cylinder Volume Formula Cylinder Formula Volume Of A Cylinder

How To Derive The Formula To Obtain The Volume Of The Frustum Of A Cone Nursing Student Tips Math Made Easy Nerd Jokes

This Page Examines The Properties Of A Right Circular Cylinder A Cylinder Has A Radius R And A Height H See Solid Geometry Volume Of A Cylinder Cylinder

Relationship Between Volume Of A Cylinder And A Sphere Youtube

Finding The Surface Area Of A Cylinder Part Of A Collection Of High School Math Bitesize Topics In One Ne Math Methods Teacher Math Resources Math Resources
How To Calculate A Derivative Of Volume Of A Cylinder Quora

Volume Of Cylinder In Terms Of Pi Formula Examples Definition
How To Calculate A Derivative Of Volume Of A Cylinder Quora

Volume Of A Hollow Cylinder Formula Definition Examples

Volume Of A Cylinder Formula Derivation Surface Area And Examples
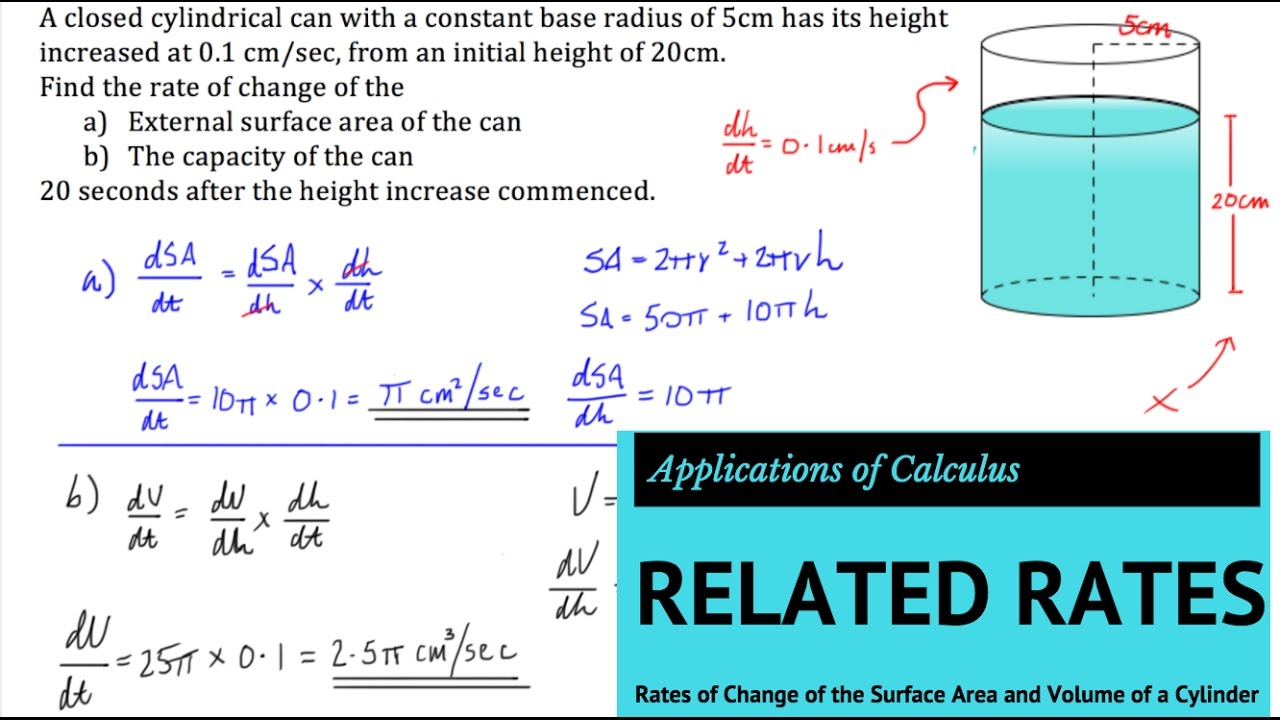
Related Rates Finding The Rates Of Change Of Surface Area And Volume Of A Cylinder Youtube

Mensuration Formula Math Formula Chart Math Formulas Formula Chart

101 Maximum Volume Of Cylinder With Surface Area 384 Pi Derivatives Calculus Youtube

Volume Of Cylinder Formula And Calculate Height Or Radius Youtube

Cylinder Inscribed In Sphere Maximum Volume Derivative Youtube

Volume Of Cylinders Cones And Spheres Review With Word Problems Word Problems Word Problem Worksheets Finding Volume

Volume Of Cylinders Cones And Spheres Coloring Activity 8th Grade Math Color Activities Volume Math
